1.2 Magnetic topological materials

Two-dimensional (2D) Kagome-lattice of $3d$ systems harbor Dirac crossings (DCs), flat bands, and electron correlations. In the intrinsic ferromagnetic (FM) Kagome lattice, the spin-orbit coupling (SOC) and the breaking of time-reversal symmetry introduce a gap at Dirac points, resulting in Chern gapped insulators. When these topological electronic states are near the Fermi level, large Berry curvatures are manifested, resulting in novel quantum properties such as the quantum anomalous Hall effect (QAHE).

Interplay between magnetism and band topology in Kagome magnets $R$Mn$_6$Sn$_6$
Y. Lee and R. Skomski and X. Wang and P. P. Orth and A. K. Pathak and B. N. Harmon and R. J. McQueeney and Liqin Ke
arxiv (2022)

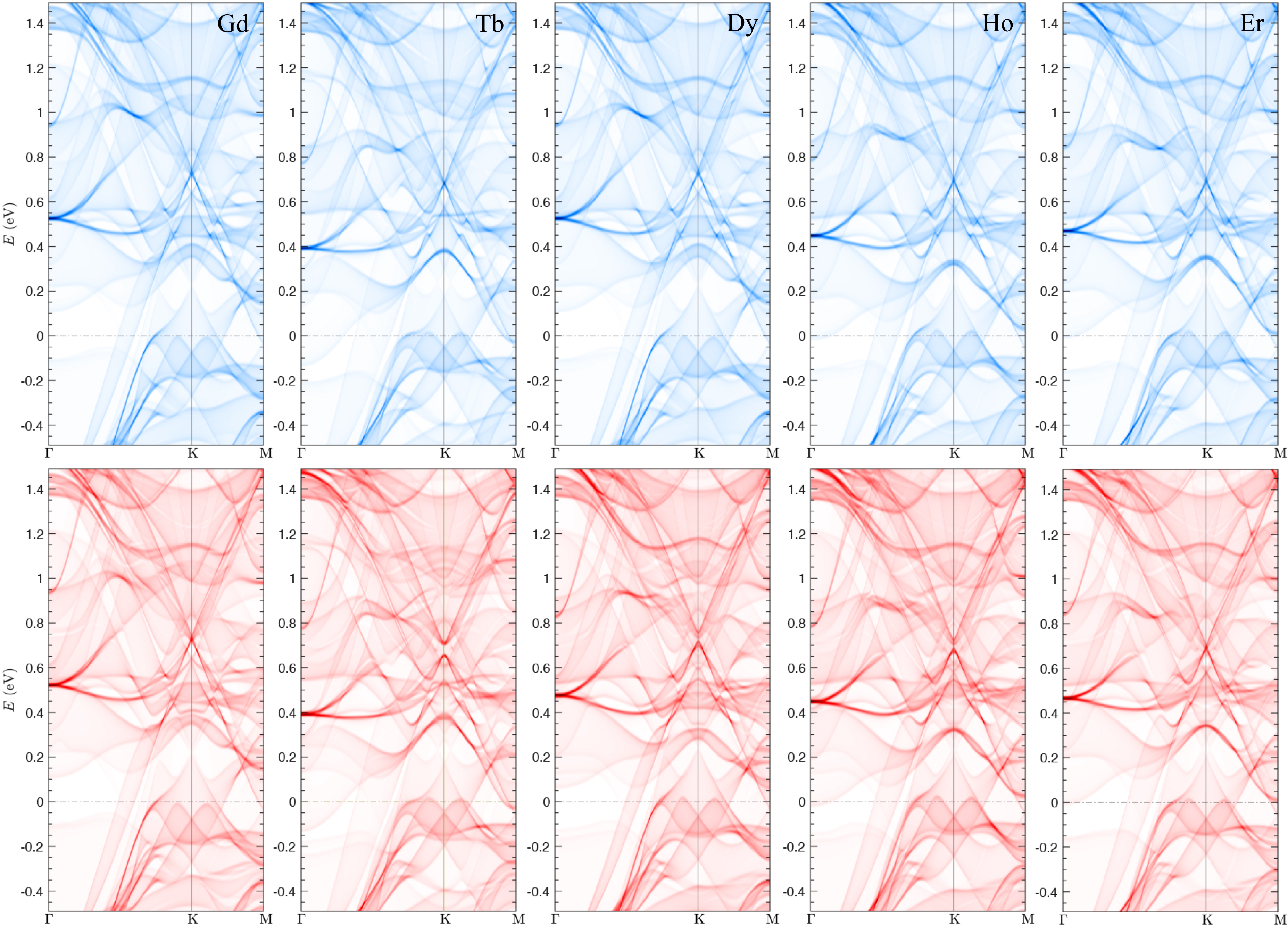
$The band structures projected on surface BZ near the Fermi level in $R$Mn$_6$Sn$_6$ with $R=$ Gd, Tb, Dy, Ho, and Er, calculated without (in blue) and with (in red) SOC. For the SOC cases, the spin quantization axis directions are set to be along the easy direction of each compound. More specifically, $\theta_\text{Gd}=90$ (Degree), $\theta_\text{Tb}=0$ (Degree), $\theta_\text{Dy}=45$ (Degree), $\theta_\text{Ho}=49$ (Degree), and $\theta_\text{Er}=90$ (Degree).$

Figure 1.2: The band structures projected on surface BZ near the Fermi level in $R$Mn$_6$Sn$_6$ with $R=$ Gd, Tb, Dy, Ho, and Er, calculated without (in blue) and with (in red) SOC. For the SOC cases, the spin quantization axis directions are set to be along the easy direction of each compound. More specifically, $\theta_\text{Gd}=90$ (Degree), $\theta_\text{Tb}=0$ (Degree), $\theta_\text{Dy}=45$ (Degree), $\theta_\text{Ho}=49$ (Degree), and $\theta_\text{Er}=90$ (Degree).

2022 (lee2022arxiv?; Riberolles et al. 2022)
2021 (Lai et al. 2021; Li et al. 2021; Riberolles et al. 2021)
2020 (Li et al. 2020)
2019 $\ge$

Publications

Lai, You, Liqin Ke, Jiaqiang Yan, Ross D. McDonald, and Robert J. McQueeney. 2021. “Defect-Driven Ferrimagnetism and Hidden Magnetization in ${\mathrm{MnBi}}_{2}{\mathrm{Te}}_{4}$.” Phys. Rev. B 103 (May): 184429. https://doi.org/10.1103/PhysRevB.103.184429.

Li, Bing, D. M. Pajerowski, S. X. M. Riberolles, Liqin Ke, J.-Q. Yan, and R. J. McQueeney. 2021. “Quasi-two-dimensional ferromagnetism and anisotropic interlayer couplings in the magnetic topological insulator ${\mathrm{MnBi}}_{2}{\mathrm{Te}}_{4}$.” Phys. Rev. B 104: L220402. https://doi.org/10.1103/PhysRevB.104.L220402.

Li, Bing, J.-Q. Yan, D. M. Pajerowski, Elijah Gordon, A.-M. Nedić, Y. Sizyuk, Liqin Ke, P. P. Orth, D. Vaknin, and R. J. McQueeney. 2020. “Competing Magnetic Interactions in the Antiferromagnetic Topological Insulator MnBi$_2$Te$_4$.” Phys. Rev. Lett. 124: 167204. https://doi.org/10.1103/PhysRevLett.124.167204.

Riberolles, S. X. M., Tyler J. Slade, D. L. Abernathy, G. E. Granroth, Bing Li, Y. Lee, P. C. Canfield, B. G. Ueland, Liqin Ke, and R. J. McQueeney. 2022. “Competing magnetic energy scales in the topological flat-band ferrimagnet TbMn$_6$Sn$_6$.” Phys. Rev. X. https://journals.aps.org/prx/accepted/66077K63Y871c500d5f54a841d69896903e91f6dc.

Riberolles, S. X. M., Q. Zhang, Elijah Gordon, N. P. Butch, Liqin Ke, J.-Q. Yan, and R. J. McQueeney. 2021. “Evolution of magnetic interactions in Sb-substituted ${\mathrm{MnBi}}_{2}{\mathrm{Te}}_{4}$.” Phys. Rev. B 104: 064401. https://doi.org/10.1103/PhysRevB.104.064401.